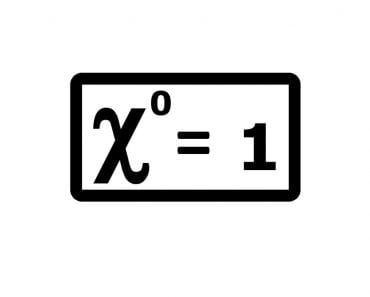An imaginary number raised to an imaginary number turns out to be real. However, while learning complex analysis, one learns that an exponential with respect to an imaginary number does not have a single, fixed value. Rather, the function is multi-valued — the value we arrived at in our calculation is just one of many values.
The imaginary unit  represents the square root of -1, such that
represents the square root of -1, such that  . Imaginary numbers live in a world of their own; the numbers are counted on an entirely different plane or axis that is solely devised for them. However, imaginary numbers have acquired a somewhat nefarious reputation, considering that their discovery has compounded the difficulty of problems that math was already replete with. I mean, as if the numbers we already had weren’t enough?
. Imaginary numbers live in a world of their own; the numbers are counted on an entirely different plane or axis that is solely devised for them. However, imaginary numbers have acquired a somewhat nefarious reputation, considering that their discovery has compounded the difficulty of problems that math was already replete with. I mean, as if the numbers we already had weren’t enough?
Our problem, however, combines not just one, but two of the many haunting aspects of mathematics. If  itself is so difficult to comprehend, what could
itself is so difficult to comprehend, what could  be? You might be surprised to know that unlike
be? You might be surprised to know that unlike  , the value of
, the value of  is a real number! How is that possible?
is a real number! How is that possible?
First, we must revise one of the most common, but least understood, mathematical operations — logarithms. Let me remind you how they work: If  , then
, then  . For instance, given that
. For instance, given that  ,
,  . Here, b is called the base of the logarithmic operation.
. Here, b is called the base of the logarithmic operation.
To solve the problem at hand, the base of our operation is  , or Euler’s number, with a value of 2.71828… If
, or Euler’s number, with a value of 2.71828… If  , then
, then  . The logarithm with the base
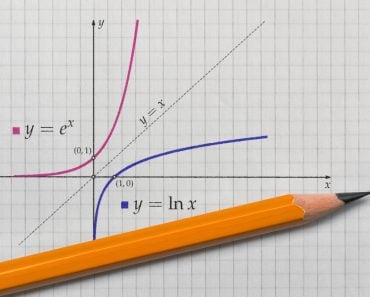
. The logarithm with the base  is known as a “natural logarithm”. It is imperative to understand that the exponential and logarithmic functions are inverse functions. This is very important for our calculation.
is known as a “natural logarithm”. It is imperative to understand that the exponential and logarithmic functions are inverse functions. This is very important for our calculation.







The second thing to recall is that  can be written as a complex number
can be written as a complex number  , which can also be written as
, which can also be written as  . However, according to Euler’s formula,
. However, according to Euler’s formula,  . Therefore,
. Therefore,  is equal to
is equal to  .
.
Now, let’s say  . Applying logarithm on both sides of the equation, we get:
. Applying logarithm on both sides of the equation, we get:




Remember that logarithmic and exponential functions are inverse functions, such that  . Thus, from the above expression, one can discern:
. Thus, from the above expression, one can discern:



Applying the exponential on both sides of the equation:

Again, we encounter the inverse functions together. They neutralize to give the outcome:





Thus, an imaginary number raised to an imaginary number turns out to be real. However, while learning complex analysis, one learns that an exponential with respect to  does not have a single, fixed value. Rather, the function is multi-valued — the value we arrived at in our calculation is just one of many values. This is because the value of
does not have a single, fixed value. Rather, the function is multi-valued — the value we arrived at in our calculation is just one of many values. This is because the value of  isn’t particularly
isn’t particularly  , but
, but  ,
,  and every odd multiple of
and every odd multiple of  . Therefore, the value of
. Therefore, the value of  also varies from
also varies from  to
to  ,
,  and so on.
and so on.
Recommended Video for you: