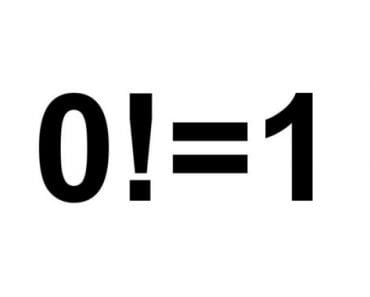Table of Contents (click to expand)
The answer is: Zero is even.
February 2, 2002 was a special day for mathematicians. When we write the celebrated date as 2002/02/02 we observe that it only contains even numbers. However, some people were incredulous, for while they were sure that the number 2 was even – as the very torch we use to illuminate other even numbers – they weren’t so sure about the “number” 0.
In a survey of 700 seven-year-old children, almost 45% of them agreed that the number 0 is, in fact, even. However, when their options were expanded to “neither odd or even”, “both odd or even” and simply “don’t know”, doubt seeped in and they reconsidered their answers. The share of people believing it to be even slipped to 32%. So, what then is 0… odd or even?
Recommended Video for you:
Technically, It’s Even
Since their inception, the distinct nature of numbers was leveraged by traders to count units of a commodity and the money it cost them. A cow or a piece of silk would be sold for some certain amount of countable shillings, but how could traders incorporate the absurd concept of zero in their transactions? How could you buy nothing? Perhaps at the cost of nothing?

This inscrutable concept exists only in abstraction, which, as some argue, doesn’t make it any different from every other number. Believed to be one of the most important discoveries in mankind’s history, it was a huge cause of discomfort initially — it is neither negative nor positive. There was no unanimity in deciding its parity – whether it was even or odd. However, it seems to pass every test that an even number must pass to prove its even-ness. So, technically, it is even. In fact, it is the most even number there is.
The very first test that comes to mind is to check whether the number is divisible by or a multiple of 2. In the past, mathematicians ascribed every even number with a degree of even-ness, which they determined by counting the number of times it could be successively divided by 2.
[adinserter block=”2″]
For instance, 14 can be divided only once for the successive number — 7 — isn’t divisible by 2. This is called a single-even. On the other hand, 12 can be divided twice, a double-even, while 24 can be split thrice, a triple-even. However, zero can be divided interminably, for when zero is divided by two, the result is again zero, which when divided by two is zero once again, a sequence that repeats forever. The calculation requires us to divide nothing or zero objects into two equal halves of nothing, which can be further divided into four equal halves of nothing or zero objects and so on.




One can also examine whether zero obeys certain fundamental arithmetic rules. For instance, the sum of two even numbers must be an even number, which is true for zero, as the sum of zero and an even number is equal to the very number added to zero – an even number. Furthermore, the sum of an odd number and an even number must be an odd number, a rule that zero also obeys, as zero added to an odd number will obviously equal the very odd number added to it! Absurd, perhaps inane, but consistent and logical, the only meritorious qualities in mathematics.
Perhaps an even simpler way is to check its position on the number line. The simplest way to determine whether a number is odd or even is to check whether it lies between two even or two odd numbers. Zero, as everyone hopefully knows, lies between -1 and 1, the progenitors of all odd numbers.